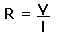Resistance and Conductance
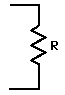
Resistance
|
The electrical resistance of a circuit component or device is defined as the ratio of the voltage applied to the electric current whichflows through it:
If the resistance is constant over a considerable range of voltage, then Ohm's law, I = V/R, can be used to predict the behavior of the material. Although the definition above involves DC current and voltage, the same definition holds for the AC application of resistors. |
Whether or not a material obeys Ohm's law, its resistance can be described in terms of its bulk resistivity. The resistivity, and thus the resistance, is temperature dependent. Over sizable ranges of temperature, this temperature dependence can be predicted from a temperature coefficient of resistance.
Resistivity and Conductivity
The electrical resistance of a wire would be expected to be greater for a longer wire, less for a wire of larger cross sectional area, and would be expected to depend upon the material out of which the wire is made. Experimentally, the dependence upon these properties is a traightforward one for a wide range of conditions, and the resistance of a wire can be expressed as
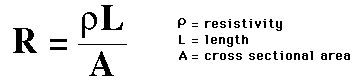
The factor in the resistance which takes into account the nature of the material is the resistivity . Although it is temperature dependent, it can be used at a given temperature to calculate the resistance of a wire of given geometry.
The inverse of resistivity is called conductivity. There are contexts where the use of conductivity is more convenient.
Electrical conductivity = σ = 1/ρ
Resistor Combinations
The combination rules for any number of resistors in series or parallel can be derived with the use of Ohm's Law, the voltage law, and the current law.
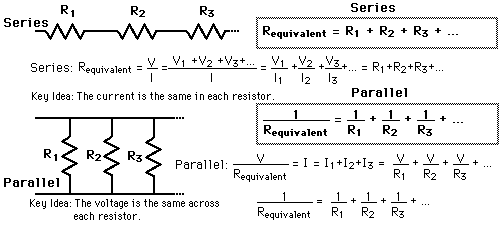
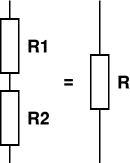
Resistors connected in Series
When resistors are connected in parallel their combined resistance is less than any When resistors are connected in series their combined resistance is equal to the individual resistances added together. For example if resistors R1 and R2 are connected in series their combined resistance, R, is given by:
Combined resistance in series: R = R1 + R2
This can be extended for more resistors: R = R1 + R2 + R3 + R4 + …
Note that the combined resistance in series will always be greater than any of the individual resistances.
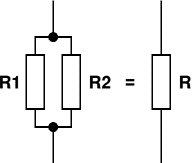
Resistors connected in Parallel
When resistors are connected in parallel their combined resistance is less than any of the individual resistances. There is a special equation for the combined resistance of two resistors R1 and R2:
|
Combined resistance of two resistors in parallel: |
R = |
R1 × R2 |
|
R1 + R2 |
For more than two resistors connected in parallel a more difficult equation must be used. This adds up the reciprocal ("one over") of each resistance to give the reciprocal of the combined resistance, R:
|
1 |
= |
1 |
+ |
1 |
+ |
1 |
+ … |
|
R |
R1 |
R2 |
R3 |
The simpler equation for two resistors in parallel is much easier to use!
Note that the combined resistance in parallel will always be less than any of the individual resistances.
Conductors, Semiconductors and Insulators
The resistance of an object depends on its shape and the material from which it is made. For a given material, objects with a smaller cross-section or longer length will have a greater resistance.
Materials can be divided into three groups:
-
Conductors which have low resistance.Examples: metals (aluminium, copper, silver etc.) and carbon.
Metals are used to make connecting wires, switch contacts and lamp filaments. Resistors are made from carbon or long coils of thin wire. -
Semiconductors which have moderate resistance.Examples: germanium, silicon.
Semiconductors are used to make diodes, LEDs, transistors and integrated circuits (chips). -
Insulators which have high resistance.Examples: most plastics such as polythene and PVC (polyvinyl chloride), paper, glass.
PVC is used as an outer covering for wires to prevent them making contact.